Research

My interests lie at the intersection of exoplanet science, instrumentation and computational imaging. Here are a few current and previous projects that I have worked on.

Differentiable coronagraph modeling for starlight subtraction

A major challenge in exoplanet imaging is to effectively remove starlight from our observations while keeping the light from the planet. Due to planets being orders-of-magnitude fainter than their host star, even small residuals of starlight can hide a planet.

In collaboration with Katie Bouman and collaborators, we are developing a forward-modeling alternative approach for starlight subtraction. See here for our first proof-of-concept paper.

I am Principal Investigator for a Cycle 4 JWST program (AR 7942) and a Cycle 5 JWST Legacy program (AR 11751) aiming to further develop and apply this framework. We have several exciting results coming up soon, so stay tuned for our future papers!

Discovery of giant planet RX J0534 b with JWST/NIRCam

I led the analysis of JWST observations that discovered and confirmed RX J0534.0-0221 b (RX J0534 b), a directly-imaged giant planet orbiting an M dwarf in Beta Pictoris. This is one of the rare giant planets directly-imaged with an M dwarf host, and (depending on how you divide it) the first one orbiting at Solar System scales (14 au) to be confirmed with common proper motion. This planet is a great laboratory to study the formation of giant planets around low-mass stars, which are typically a challenging outcome of planet formation. You can read the paper here.

Imaging sub-Jupiters for the first time with JWST/NIRCam

JWST is the only observatory for the foreseeable future that will be able to directly image wide-separation, sub-Jupiter mass exoplanets. Its extraordinary near-infrared and mid-infrared sensitivity make it ideal to discover and characterize the atmospheres of young, low mass giant planets.

I am leading the systematic analysis of more than 100 JWST/NIRCam coronagraphic observations aiming to find the lowest mass planets directly imaged to date (PIs: Aarynn Carter and Beth Biller). The first in a series of papers with our results can be found here, where we look at the planet-disk system TWA 7.

Biases in orbit-fitting of directly-imaged exoplanets

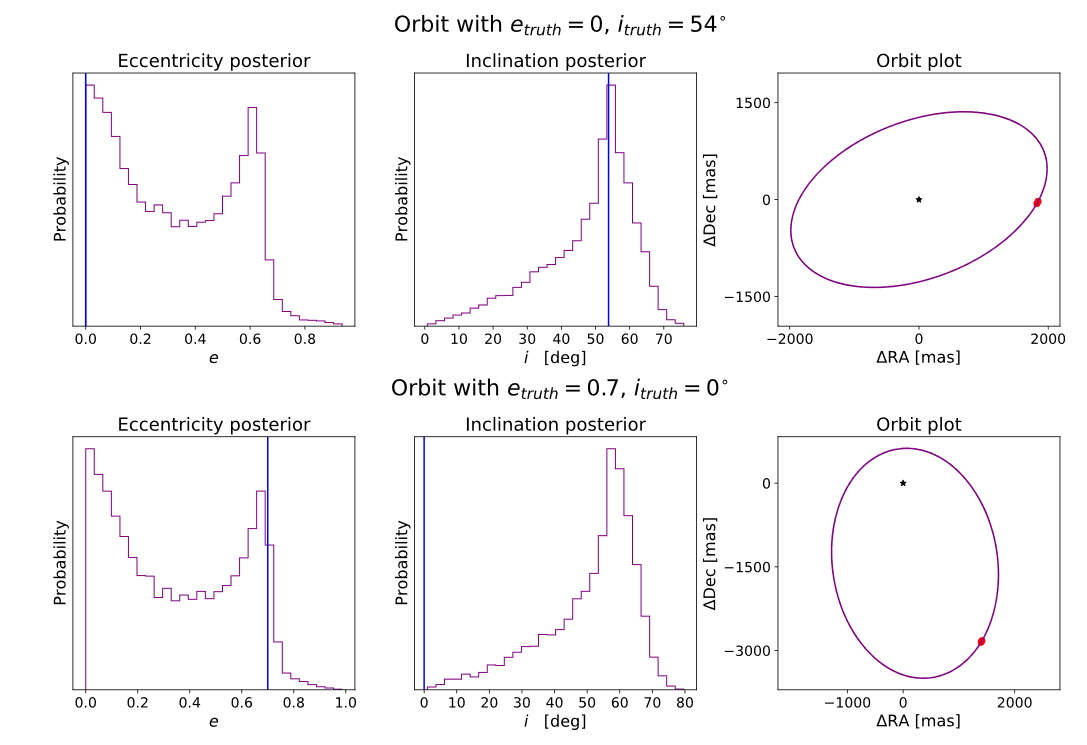
Direct-imaging is most sensitive to planets very far from their star, where they are easier to detect. Given that the orbital period of a planet increases as its semi-major axis increases (by Kepler's third law), directly-imaged planets tend to have relatively long periods, some even up to hundreds or thousands of years. This means that even with years of observations, we often times can see the planet only moving through a small fraction of its orbit, making the inference of the orbit of the planet very challenging.

Given that reliably fitting the orbit of a planet can reveal vital information about its formation, history and environment, in collaboration with Jason Wang and Sarah Blunt I carried out a systematic statistical analysis of the biases we are most prone to when estimating orbits of directly-imaged planets. Performing our analyses with widely-used Bayesian frameworks and setups, we characterize biases such as the eccentricity-inclination degeneracy and the coverage of the credible intervals over orbital parameters. See here for our paper.